TS 10th Chapter 4 Pair of Linear Equations in Two Variables
TS 10th Chapter 4 Pair of Linear Equations in Two Variables, Parallel, coincident, and intersecting lines. Dependent and independents lines
An equation of the form ax + by + c = 0 where a, b, c are real numbers and a2 + b2 ≠ 0 is called a linear equation in two variables x and y.
Two linear equations in the same variables are called a pair of linear equations in two variables
a1x + b1y + c1 = 0; a2x + b2y + c2 = 0 are the pair of linear equations in two variables in x and y.
Solution of Pair of linear equations in two variables:
For linear equations in two variables, there are infinitely many solutions.
Ex: x + y = 10
x =7, y = 3; x = 6, y = 4; x = 1, y = 9; x =2, y = 8; x=3, y = 7 like we have infinitely many solutions.
⇰ For finding exact values of x and y we have to know two linear equations.
⇰ A pair linear equations in two variables solved by four methods
- Graphical method
- Substitution method
- Elimination method
- Cross – Multiplication method
Solving the pair of linear equations in two variables by using graphical Method:
1. 2x + y −5 = 0, 3x – 2y − 4 = 0
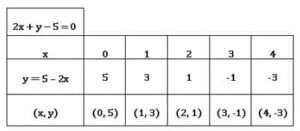
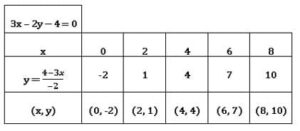

After plotting the points in the above tables in Cartesian plane, we observe that two straight lines intersect at the point (2, 1)
There is only one solution for this pair of linear equations in two variables.
These equations are known as consistent pair of linear equations and they have a unique solution.
2. 2x – 3y = 15; 4x – 6y = 9
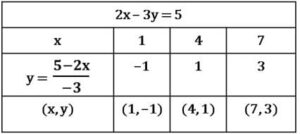
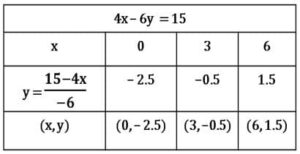

After plotting the points in the above tables in Cartesian plane, we observe that two straight lines are parallel
There is no solution for this pair of linear equations in two variables.
These equations are known as inconsistent pair of linear equations and they have no solution.
3. 3x + 4y = 2; 6x + 8y = 4


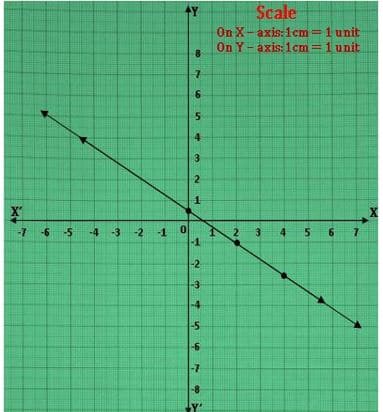
After plotting the points in the above tables in Cartesian plane, we observe that two straight lines are coincide
There are infinitely many solutions for this pair of linear equations in two variables.
These equations are known as consistent pair of linear equations and they have infinitely solution.
Consistent and inconsistent:
If the system of equations has a solution, then they are consistent. If the system of equations has no solution, then they are inconsistent.
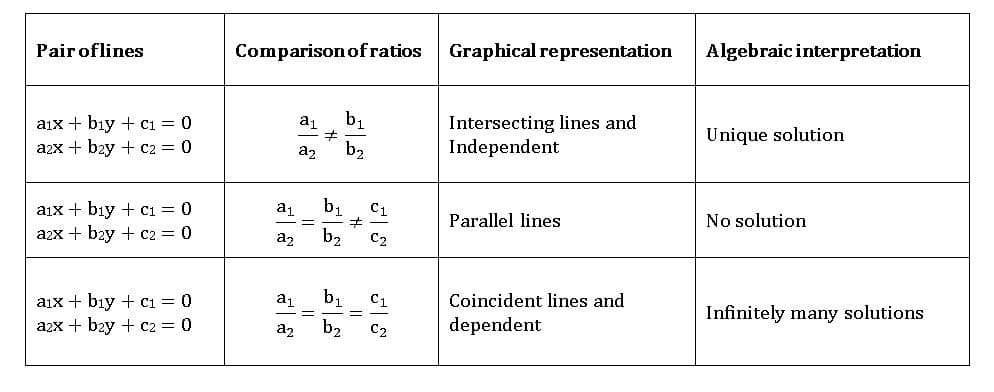
The relationship between coefficients and the nature of the equation system:
Examples:
1. Draw the graph of the following pair of linear equations in two variables and find their solution from the graph
3x – 2y = 2 and 2x + y = 6
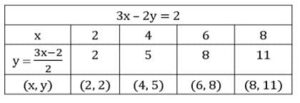

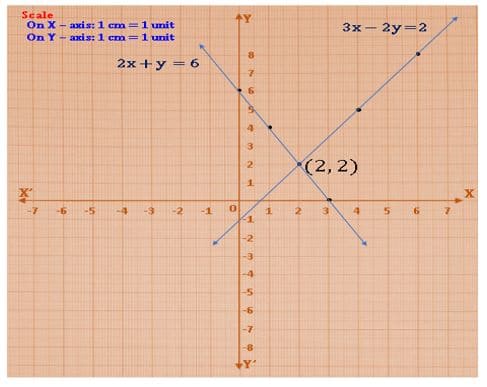
The two lines intersect at the point (2, 2)
∴ solutions is x = 2, y = 2
2. Draw the graph of the following pair of linear equations in two variables and find their solution from the graph
x – 2y = –1 and 2x – y – 4 = 0
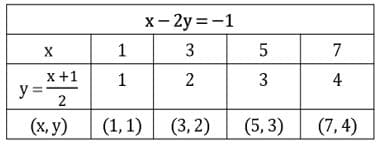
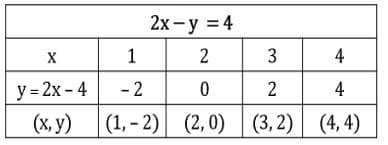
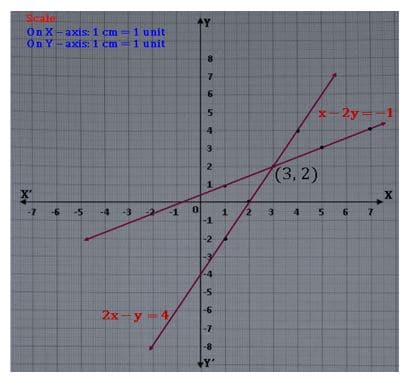
The two lines intersect at the point (3, 2)
∴ solutions is x = 3, y = 2
3. Represent the solution of linear equation graphically
x – 2y = –3 and 2x + y = 4
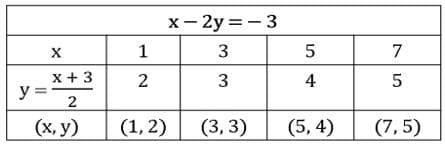

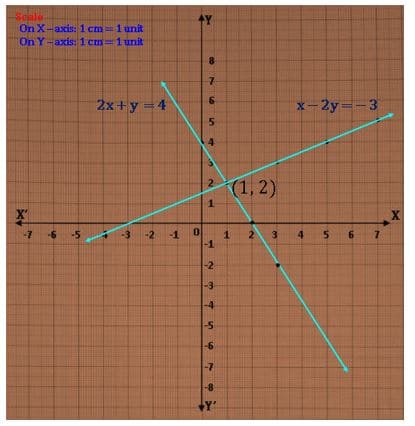
The two lines intersect at the point (1, 2)
∴ solutions is x = 1, y = 2
Word problems:
1. Neha went to a sale to purchase some points and skirts. When her friend asked her how many of each she had bought, she answered “The number of skirts is two less than twice the number of points purchased. Also, the number of number of skirts is four less than four times the number of pants purchased”.
Help her friend to find how many pants and skirts Neha bought.
Sol:
Let the number of points = x
and the number of skirts = y
the number of skirts is two less than twice the number points purchased
⟹ y = 2x – 2
the number of number of skirts is four less than four times the number of pants purchased
⟹ y = 4x – 4
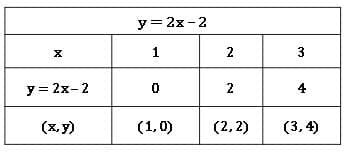
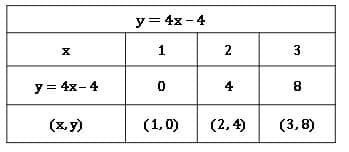
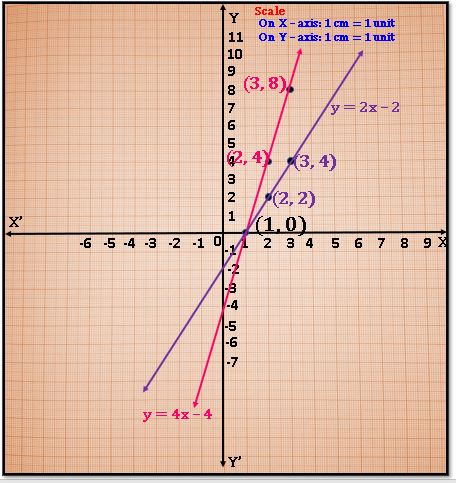
The two lines intersect at the point (1, 0)
∴ solution is x = 1, y = 2
No. of Points = 1 and no. of skirts = 0
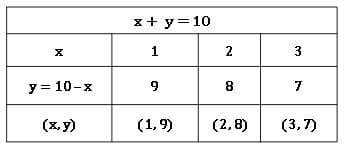
2. 10 students of a class X took part in a Mathematics quiz. If the no. of girls is 4 more than the no. of boys, then find the no, of boys and no. of girls who took part in the quiz.
Sol:
let the no. of boys = x
No. of girls = y
Total no. of students = 10
⟹ x + y = 10
The no. of girls is 4 more than the no. of boys
⟹ y = x + 4
⟹ x – y = – 4
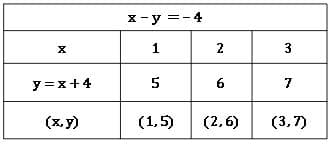
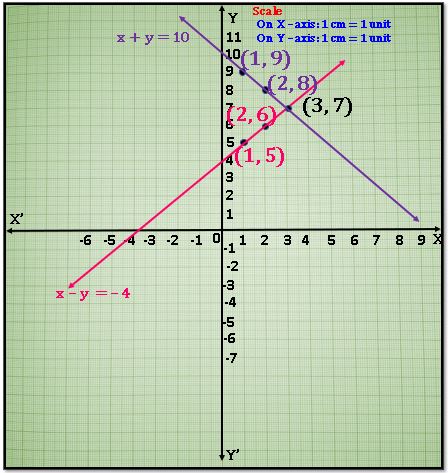
The two lines intersect at the point (3, 7)
∴ solution is x = 3, y = 7
No. of boys = 3 and no. of girls = 7
3. Half the perimeter of a rectangular garden, whose length is 4m more than its width is 36 m. Find the dimensions of the garden.
Sol:
let the width of garden = x m.
Length of garden = y m.
Length of garden is 4m mote than its width
⟹ y = x + 4
x – y = – 4
half of the perimeter of rectangular garden is 36 m.
⟹ x + y = 36
⟹ x – y = – 4
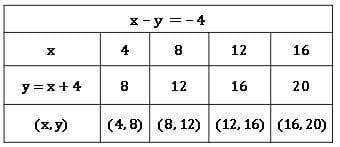


The two lines intersect at the point (16, 20)
∴ solution is x = 16, y = 20
Width of garden = 16 m and Length of garden = 20 m
4. The area of a rectangle gets reduced by 80 sq units if its length is reduced by 5 units and breadth is increased by 2 units. If we increase the length by 10 units and decrease the breadth by 5 units, the area will be increased by 50 sq units. Find the length and breadth of rectangle.
Sol:
Let the length of rectangle = x units
the breadth of rectangle = y units
The area of rectangle = xy sq units
If the Length of rectangle is reduced by 5 units and breadth is increased by 2 units, then area will be reduced by 80 sq units.
⟹ (x – 5) (y + 2) = xy – 80
⟹ xy + 2x – 5y – 10 = xy – 80
⟹ 2x – 5y = – 70
If length is increased by 10 and breadth decreased by 5 then area will be increased by 50 squnits
⟹ (x + 10) (y – 5) = xy + 50
⟹ xy – 5x + 10y – 50 = xy + 50
⟹ – 5x + 10y = 100

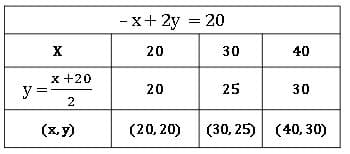
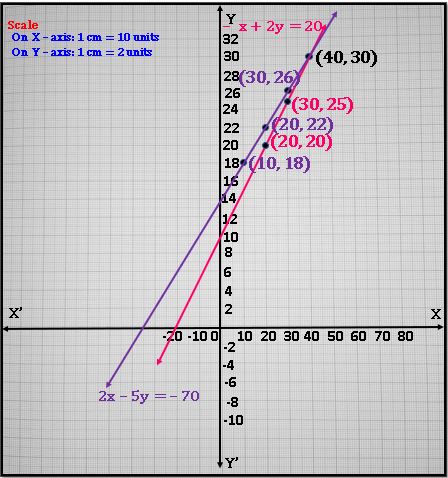
The two lines intersect at the point (40, 30)
∴ solution is x = 40, y = 30
Length of rectangle = 40 units and Breadth of rectangle = 30 units
5. In X class, if three students sit on each bench one student will be left, if four students sit on each bench, one bench will be left. Find the number of students and number of benches in that class.
Sol:
Let the no. of students = x
The no. of benches = y
If three students sit on each bench one student will be left
⟹ 3y = x – 1
⟹ x – 3y = 1
If four students sit on each bench one bench will be left
⟹ 4 (y – 1) = x ⟹ 4y – 4 = x
⟹ x – 4y = – 4
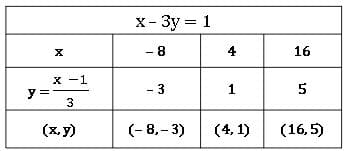
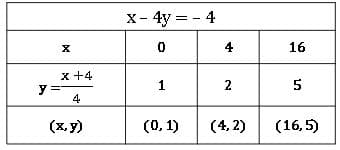

The two lines intersect at the point (16, 5)
∴ solution is x = 16, y = 5
No. of students = 16 and No. of benches = 5
The relationship between coefficients and the nature of the equation system Related Problems:
1. Check whether the following pair of linear equations have a unique solution, infinitely many solutions or no solution
(i) 2x + 3y = 1; 3x – y = 7
(ii) 2x + 3y = 5; 4x + 6y = 10
(iii) 5x + 3y = 4;10x + 6y = 12
Sol:
(i) Given equations are x + 3y = 1; 3x – y = 7
a1 = 1, b1 = 3, c1 = 1; a2 = 3, b2 = –1, c2 = 7

∴ Given equations have unique solution
(ii) Given equations are 2x + 3y = 5; 4x + 6y = 10
a1 =2, b1 = 3, c1 = 5; a2 = 4, b2 = 6, c2 = 10

∴ Given equations have infinite solutions
(iii) Given equations are 5x + 3y = 4;10x + 6y = 12
a1 =5, b1 = 3, c1 = 4; a2 = 10, b2 = 6, c2 = 12
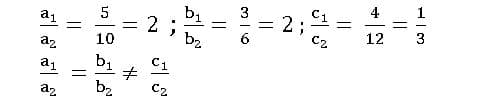
∴ Given equations have no solution
2. For what value of ‘p’ the following pair of equations has a unique solution
2x + py = – 5 and 3x + 3y = – 6
Sol:
Given equations are 2x + py = – 5, 3x + 3y = – 6
a1 =2, b1 = p, c1 = –5; a2 = 3, b2 = 3, c2 = –6
Above equations have a unique solution
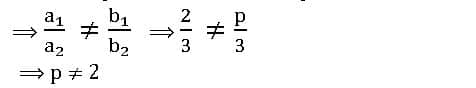
∴ Given pair of linear equations have a unique solution when p ≠ 2
3. For what value of ‘p’ the following pair of equations has a unique solution
px + 3y –(p – 3)= 0 and 12x + py – p = 0
Sol:
Given equations are px + 3y – (p – 3) = 0, 12x + py – p = 0
a1 =p, b1 = 3, c1 = – (p – 3),; a2 =12, b2 = p, c2 = –p
Above equations have infinitely many solution

∴ Given pair of linear equations have infinitely many solutions when p = ±6
4. For what value of ‘k’ the following pair of equations represent coincident lines.
3x + 4y + 2 = 0 and 9x + 12y + k = 0
Sol:
Given equations are 3x + 4y + 2 = 0 and 9x + 12y + k = 0
a1 =3, b1 = 4, c1 = 2; a2 = 9, b2 = 12, c2 = k
Above equations are coincident lines
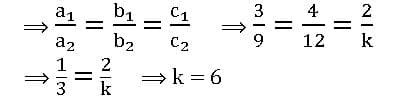
∴ Given pair of linear equations are coincident when k = 6
5. For what value of ‘k’ the following pair of equations represents the parallel lines
2x – ky + 3 = 0 and 4x + 6y – 5 = 0
Sol:
Given equations are 2x – ky + 3 = 0, 4x + 6y – 5 = 0
a1 =2, b1 = –k, c1 = 3; a2 = 4, b2 = 6, c2 = –5
Above equations represents parallel lines
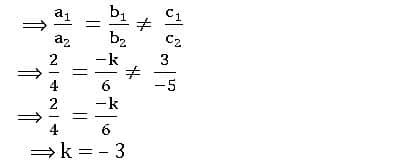
∴ Given pair of linear equations represents parallel lines when k = – 3
Substitution Method:
In this method, we make one of the variables x or y as the subject from the 1st equation (or 2nd equation). Substitute this equation in 2nd equation (or 1st equation) and get the value of the variable involved, then by substituting this value in any one of the equations we get the value of second variable.
1. Solve the following pair of equations by using substitution method
2x – y = 5 and 3x + 2y = 11
Sol:
Given equations are 2x – y = 5 ———— (1)
3x + 2y = 11———– (2)
From (1)
y = 2x – 5
substitute y value in (2)
⟹ 3x + 2(2x – 5) = 11
3x + 4x – 10 = 11
7x = 11 + 10 = 21
x = 3
now y = 2x – 5
⟹ y = 2(3) – 5 = 6 – 5 = 1
∴ the solution is x = 3 and y = 1
2. Solve the following pair of equations by using substitution method
2x + 3y = 9 and 3x + 4y = 5
Sol:
Given equations are 2x + 3y = 9 ———— (1)
3x + 4y = 5———– (2)
From (1)
3y = 9 – 2x

∴ the solution is x = – 21 and y = 17
3. Solve the following pair of equations by using substitution method
3x – 5y = – 1 and x – y = – 1
Sol:
Given equations are 3x – 5y = – 1 ———— (1)
x – y = – 1———– (2)
From (2)
y = x + 1
substitute y value in (1)
⟹ 3x – 5(x + 1) = – 1
3x – 5x – 5= – 1
– 2x – 5= – 1
– 2x = – 1 + 5
– 2x = 4 ⟹ x = – 2
now y = x + 1
⟹ y = – 2 + 1 = – 1
∴ the solution is x = – 2 and y = – 1
4. Solve the following pair of equations by using substitution method
x + = 6 and 3x – = 5
Sol:
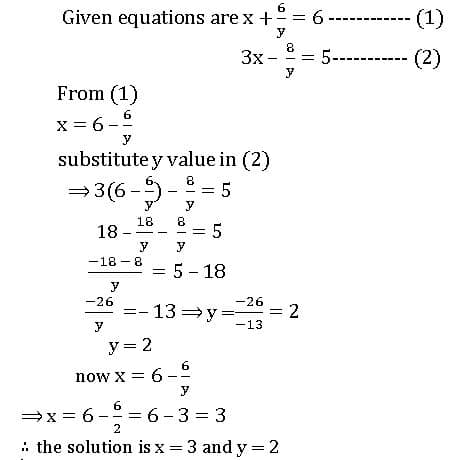
5. Solve the following pair of equations by using substitution method
0.2x + 0.3y = 1.3 and 0.4x + 0.5y = 2.3
Sol:


Elimination Method:
In this method first we eliminate one of the two variables by equating its coefficients. This give a single equation which can be solved to get the value of the other variable
Steps for Elimination Method:
- Write the both the equations in the form of ax + by = c
- Multiply both the equations by suitable non-zero real number to make the coefficient of one variable (x or y) equal
- If the variable to be eliminated has the same sign in both the equations, subtract one equation from another to get an equation in one variable.
- Solve the equation for the one variable
- Substitute the value of this variable in any one of the given equations and find the value of the eliminated variable.
1. Solve the following pair of equations by using Elimination method
2x – y = 5 and 3x + 2y = 11

2. Solve the following pair of equations by using the Elimination method
8x + 5y = 9 and 3x + 2y = 4
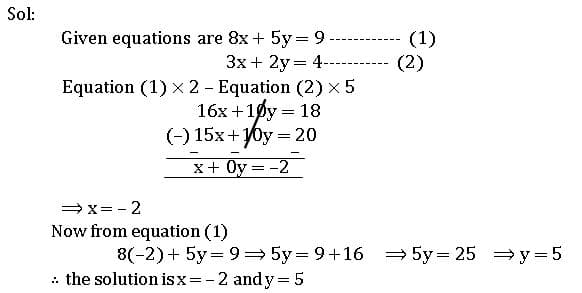
3. Solve the following pair of equations by using the Elimination method
3x + 4y = 25 and 5x – 6y = –9
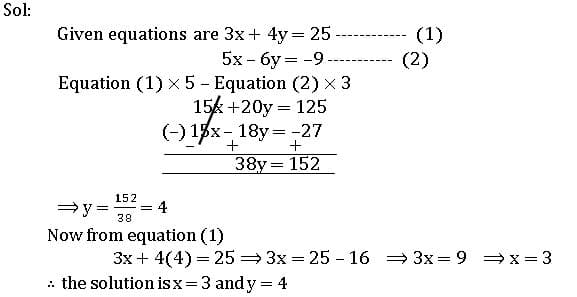
4. Solve the following pair of equations by using the Elimination method
2x + y = 5 and 3x – 2y = 4

5. Solve the following pair of equations by using Elimination method
3x + 2y = 11 and 2x + 4y =4
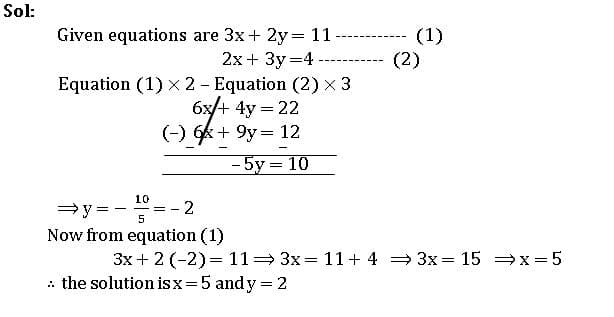
Visit my Youtube Channel: Click on Below Logo




